Infinity
Where Do You Stop? Chapter 7:
She paused for just a moment, as if she were gathering her thoughts, but a glazed, ecstatic look came over her eyes, and when she began to speak, her voice had in it a breathless tremor that I recognized: she was thrilled.
“Infinity is really anything that is not finite,” she said, “but I know that doesn’t seem like much of an answer. In this case, you can think of infinity as a place so far away that it can never be reached. We can approach it, but we can never reach it. In the most general sense, infinity is any limit we can approach but can never reach.”
Infinity is something which is boundless, endless, or larger than any natural number. It is often denoted by the infinity symbol ∞.
Since the time of the ancient Greeks, the philosophical nature of infinity was the subject of many discussions among philosophers. In the 17th century, with the introduction of the infinity symbol and the infinitesimal calculus, mathematicians began to work with infinite series and what some mathematicians (including l’Hôpital and Bernoulli) regarded as infinitely small quantities, but infinity continued to be associated with endless processes. […] At the end of the 19th century, Georg Cantor enlarged the mathematical study of infinity by studying infinite sets and infinite numbers, showing that they can be of various sizes. For example, if a line is viewed as the set of all of its points, their infinite number (i.e., the cardinality of the line) is larger than the number of integers. […]
In physics and cosmology, whether the Universe is spatially infinite is an open question.
Universe, The: Bounded (Closed, Finite) or Unbounded (Open, Infinite)?
Where Do You Stop? Chapter 7:
“I used to love to think about infinity when I was a girl,” she said, as if recalling an old boyfriend. “My friends and I used to lie on our backs and look at the stars and wonder how big the universe was and what was at the edge of it. The universe isn’t infinite, though, Matthew. Of course, it’s hard to say just how extensive it is, especially since space—well, space-time really, but we’ll get into that later in the year—is probably curved, […] Anyway, these are some of the Big Questions, and Big Questions like these are what we’re going to be exploring together this year.”
[If we are meant to consider Peter and Kraft exact contemporaries (and I happen to know that we are), then the science that Miss Rheingold presents to the class is more than 65 years old. I tried to find a general science textbook of that vintage, but failed. The following is excerpted from an article posted online in 2001.]
One of the most profound insights of General Relativity was the conclusion that mass caused space to curve, and objects travelling in that curved space have their paths deflected, exactly as if a force had acted on them. If space itself is curved, there are three general possibilities for the geometry of the universe. Each of these possibilities is tied to the amount of mass (and thus to the total strength of gravitation) in the universe, and each implies a different past and future for the universe.
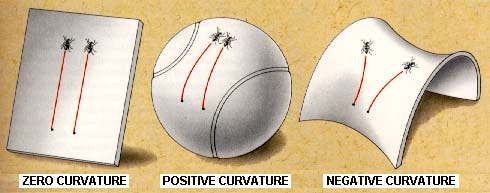
First, let’s look at shapes and curvatures for a two-dimensional surface. Mathematicians distinguish 3 qualitatively different classes of curvature, as illustrated in the following image:
The flat surface at the left is said to have zero curvature, the spherical surface is said to have positive curvature, and the saddle-shaped surface is said to have negative curvature.
The preceding is not too difficult to visualize, but General Relativity asserts that space itself (not just an object in space) can be curved, and furthermore, the space of General Relativity has 3 space-like dimensions and one time dimension, not just two as in our example above. This IS difficult to visualize! Nevertheless, it can be described mathematically by the same methods that mathematicians use to describe the 2-dimensional surfaces. So what do the three types of curvature—zero, positive, and negative—mean to the universe?
If space has negative curvature, there is insufficient mass to cause the expansion of the universe to stop. In such a case, the universe has no bounds, and will expand forever. This is called an open universe.
If space has no curvature (i.e, it is flat), there is exactly enough mass to cause the expansion to stop, but only after an infinite amount of time. Thus, the universe has no bounds and will also expand forever, but with the rate of expansion gradually approaching zero after an infinite amount of time. This is termed a flat universe or a Euclidian universe (because the usual geometry of non-curved surfaces that we learn in high school is called Euclidian geometry).
If space has positive curvature, there is more than enough mass to stop the present expansion of the universe. The universe in this case is not infinite, but it has no end (just as the area on the surface of a sphere is not infinite but there is no point on the sphere that could be called the “end”). The expansion will eventually stop and turn into a contraction. Thus, at some point in the future the galaxies will stop receding from each other and begin approaching each other as the universe collapses on itself. This is called a closed universe.
Have you missed an episode or two or several?
You can begin reading at the beginning or you can catch up by visiting the archive or consulting the index to the Topical Guide. The Substack serialization of Little Follies begins here; Herb ’n’ Lorna begins here; Reservations Recommended begins here; Where Do You Stop? begins here.
You can listen to the episodes on the Personal History podcast. Begin at the beginning or scroll through the episodes to find what you’ve missed. The Substack podcast reading of Little Follies begins here; Herb ’n’ Lorna begins here; Reservations Recommended begins here; Where Do You Stop? begins here.
You can listen to “My Mother Takes a Tumble” and “Do Clams Bite?” complete and uninterrupted as audiobooks through YouTube.
You can ensure that you never miss a future issue by getting a free subscription. (You can help support the work by choosing a paid subscription instead.)
At Apple Books you can download free eBooks of Little Follies, Herb ’n’ Lorna, and Reservations Recommended.
You’ll find overviews of the entire work in An Introduction to The Personal History, Adventures, Experiences & Observations of Peter Leroy (a pdf document) and at Encyclopedia.com.